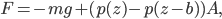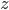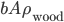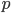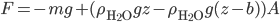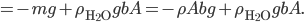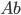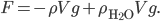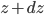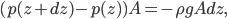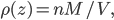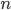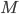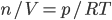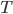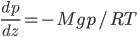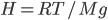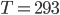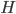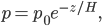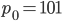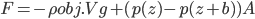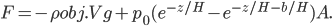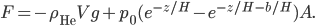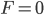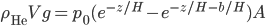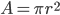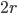Boats, hot air balloons, helium balloons: all of these things defy gravity. Gravity is pulling down on them, but they don't fall all the way to the ground. If you had a helium balloon on the moon, it would not rise: gravity would prevail and pull the balloon to the ground. That's what gravity does. So how is it that things float, and to what extent will they do so? Well, obviously it has something to do with them being in air or water. But why?
Let's start out with something a bit easier. When you swim to the bottom of a pool that's more than, say, 6 ft deep, your ears hurt because the pressure (hydrostatic pressure) is larger there than we're used to. Why is that? Because the water at the bottom of the pool has to hold up all the water that's above it. And water weighs a lot!
If you have glass, and you add a layer of water to it, the molecules in that layer are at a certain density. When you add a 2nd layer, the 1st layer gets crunched down a bit due to the weight of the water in the 2nd layer. It doesn't compress very much in terms of volume, but the pressure rises until it's holding the 2nd layer up. As you add more layers, the 1st layer gets squished more and more, and its pressure keeps going up. The pressure in the 2nd layer also goes up as it has to hold the 3rd, 4th, 5th layers up as well. Think of it like gymnasts forming a column, with each guy standing on a lower guy's shoulders. The more guys in the column, the more stress on the first guy.
This implies that there's a gradient of pressure: the lowest layer has the highest pressure, the highest layer has the lowest pressure, and there's a linear relationship between depth and pressure. The molecules at the bottom have to crash into the molecules above them harder in order to hold the weight up. The weight is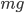 
, but it's better to express the mass as the density, mass divided by volume, times the volume. So the pressure is
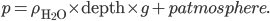
is the density of water. That's simple, because the density of water doesn't depend very much on depth, and is therefore just a constant. So the pressure at 2 meters is 
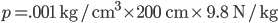which is 1.960 N/cm2 or 19600 pascals more than at sea level. That's about a 20% increase, since normal atmospheric pressure is 101000 pascals. And your ears aren't really "designed" to handle that.
Now, some things float and some things sink in water. Things that are "lighter" than water float. Wood, ice, (apples, churches, very small rocks) and other things that are less dense float. We want to say why that is.
Let's just focus on a block of wood, submerged in water, that has a thickness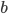 
and area 
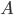. Its density is 
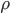. The pressure on the bottom of it is higher that the pressure on the top of it, for the simple reason stated above.
Important!
So the net force on it is
where
is the depth of the top edge. The mass of the wood is 
. Since we already know 
,
The volume is
, so
If the density
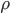is bigger than , the object has a net force downward and therefore sinks. But if 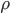is less than , which for wood, it is, the object actually has a net force upward, and rises. Density is the determiner. The buoyant force, upwards, is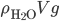 
, which is the weight of the water that would have been in that volume if the wood wasn't there. This is called Archimedes' principle, about which he supposedly screamed "Eureka"' and ran naked through the streets of Athens. If the buoyant force is greater than the weight, you have floating.
Now, the water at a certain depth is holding up the water above it, but it's also holding up the entire atmosphere above it too. That's why the pressure is 
more than atmosphere. At a depth of zero the pressure is regular atmospheric pressure 101 kPa. Things also have buoyancy in air. Helium balloons float because they are "lighter than air". But lighter than air means less dense.
Just as before, let's determine how pressure varies with height, but this time in the air. It will be a bit different physics this time, since the density of air is not constant with altitude. In fact, the air gets thinner as you go up.
Important!
Imagine a volume of air with area and height 
, at an altitude from to 
. The force of gravity pulling it down is equal to the difference in pressure on both sides. So
where
is the density of air. Divide by :
The thing is,
also depends on .
where
is the number of moles and 
is the mass per mole. From the ideal gas law, we know 
. Now, presuming that 
doesn't vary with ,
and thus
Of course, it's a pretty dubious assumption that
stays the same! However, pressure varies much more quickly than temperature, so don't worry about it for now.
Anyway, call 
the scale height, so that (with average molar mass of dry air 28.97 g/mol and taking 
K) 
is about 8.6 kilometers (5.3 miles). That means that every 5.3 miles the pressure drops by a factor of about 2.71. The expression of pressure is now
where
kPa is pressure at sea level. This isn't perfect, but it's alright. So now for the same block of some object, which has density 
, the total force is
and
Unlike for water, the ability to float in air depends upon elevation. Duh! Once you get high enough that there's no air, the air can't provide buoyancy.
For relatively low altitudes, the function 
looks almost exactly like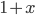 
, so this is approximately
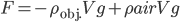
which is the same as Archimedes' principle; that is, the buoyant force in air is approximately equal to the weight of air displaced.
But for high altitudes, we can't make that assumption. So we can ask another question: when will a helium balloon stop floating? This is just meant to give us an idea, so it won't be totally accurate.
Important!
We just need to calculate
We want to know where these forces balance, which is when
.
Ok, let's solve for
. What, you can't do it? Yeah, this equation cannot be solved algebraically. The best thing to do is to plug in all of the numbers and solve it numerically. That is, you have a computer check values for until the equation is satisfied. We'll assume a spherical balloon of 1 m diameter filled with helium, and neglect the mass of the balloon itself. Then, the area 
and is roughly 
. We'll take 
K.Then, the height that the balloon rises to is roughly 20 km or 12 miles. At that point, the weight of the object balances the weakened buoyant force, and in principle it will just stay there forever. But this inherently limits our ability to survey the Earth by balloon. We can probably never get to the outer layer of atmosphere, called the thermosphere, by use of a balloon. For that we have to use rockets to position satellites, since buoyancy peters out too fast as you go away from the ground.
Footnote: There are several technical problems with this final calculation. For very light material of the balloon, the pressure inside will expand the balloon as it goes up, due to the reduced pressure outside with constant pressure inside. The balloon will therefore pop before too long. On the other hand, if you used a hard material, it would be considerably heavier, and therefore you can't ignore the weight of the balloon itself. Real weather balloons can reach much higher than this (almost 100 miles), whereas a regular rubber balloon might only make it a few hundred feet before bursting.