Thermo for Normals, Parts 1 and 2 (of 30)

Thermo for Normals is a short book (~100 pages) I wrote that conveys the basics of thermodynamics without all of the mathematical complexity. Over the next year I will be posting 1 or 2 sections of the text per week. I don't know whether I will ever try to publish it in any other form, though perhaps as an Amazon e-book it wouldn't be a bad idea. Questions, comments, criticisms, and corrections are welcome.

Introduction

Thermodynamics is the one topic in physics that everyone should know. Our world is filled with thermal phenomena, from the air we breathe, whirring fans in our computers, the passing of high pressure weather systems, or just the simple act of putting cream into a cup of coffee. Engines, generators, refrigeration, and the like drove the industrialization of the world in the past century. And we now face the calamity of a warming planet, driven by a marginal increasing imbalance of heat from the Sun and Earth's ability to get rid of it.

People don't seem very interested in this topic. It doesn't make it into Star Trek episodes or into popular non-fiction books like A Brief History of Time. This could be because people just don't see the need to analyze things that are perceived to be commonplace—ordinary. Water boils when you apply heat for long enough. It's cold in the mountains. So what?

And yet, all physics is thermodynamics! Any phenomenon of interest in the real world is one made up of a huge amount of particles. Saying something meaningful about this is to describe our universe. If physical laws are the way the chess pieces move, thermodynamics is the game of chess itself.

I think that many people probably think that thermo is a hard topic. In reality, all physics is hard if you try to get all the details right; the universe is ugly, messy, and complicated. But lots of people learn, in either high school or college, the introductory mechanics material. Can they do everything? No. But they can learn something about the world. They cover the 3 laws of motion, the conservation laws, and then do some problems to see where these laws come into play in the real world.

Most people can name 2 out of 3 of Newton's Laws. Most people cannot name a single one of the laws of thermodynamics in any form.

And the fact of it is that you already know a lot about thermodynamics. Though you may not realize it immediately. And this is a huge benefit: most of physics depends on intuition about what should happen. After that, you just need to apply that intuition to work out the quantities. (This is why quantum mechanics is so hard...none of us has any intuition about that!)

This is not meant to be "Thermodynamics for Dummies". I don't think a dummy can learn thermo. I do think that anyone with a Bachelor's degree can, and probably a lot of people with a high school diploma too. The level of physics knowledge required to read the main body of this series will be minimal. A 1 year high school course or a one term college class is more than enough.

Now about math. First of all, I want to make clear that all the math is my job. At no point do you need to reason your way through some math to understand something. You don't even necessarily have to follow along. In fact, I've sectioned off all of it in boxes like this:

If you don't want to read it, don't! These calculations are as simple as they can be, but they are honest physics calculations, and therefore do contain some calculus.

Now, that said, sometimes the easiest way to say something is to show an equation. Imagine if every time I wanted to say I had to say "well, we know that force equals mass times acceleration". As long as you can recognize what the operations of addition, subtraction, multiplication, division, and taking something to a power, you'll be fine. You should be familiar with these things, though:

• How to read a graph, or a chart
• numbers expressed in scientific notation, or as a number times a power of 10
• What a unit of measurement is

If you know what a chart means, if you understand 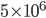is 5 million, and if you know what a meter is, you'll be alright.

This series will proceed as follows. First we'll review the laws of mechanics, including Newton's laws of motion, energy conservation, and momentum conservation. That's how the chess pieces move. Next, we'll take a look at some common thermodynamic phenomena that can be understood with only this basic mechanics understanding. After that, we'll come to the 3 laws of thermodynamics themselves, delving into their consequences.

Here are some phenomena that I'd like to address. It is not comprehensive, but just a few examples:

• Why do puddles dry up if they aren't boiling?
• How do you make "cold"?
• Why is it cold in the mountains?
• Why can't you make good tea in Colorado?
• Should you leave the heat on when you leave the house?
• How energy efficient can cars be made?
• How does global warming work? Why can't we just air condition the Earth?
• What does thermodynamics suggest about the beginning and end of the universe?

We'll try to learn from the bottom up, starting with things that we all see and know every day, and then getting a bit more technical to try to wrestle with stuff that isn't so commonplace.

List of Terms

Here are some words that we need to bandy about that I do not define elsewhere in the text.

Atoms - The building block of all normal matter. The atom has a very small center, called the nucleus, made up of extremely heavy protons (positively charged) and neutrons (no charge). Surrounding this nucleus are very light, negatively charged particles called electrons.

Macroscopic - Something that's average, everyday life-sized. Air in the room, baseballs, bottles of water, these are all macroscopic. By contrast, atoms or the transistors on microchips are microscopic.

Moles - A mole is just a word that means 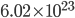of something. If you have 1 mole of argon gas, that means you have 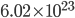argon atoms.

Molecule - One or more atoms that are bonded together. Hydrogen gas is molecular because it is almost always , or two hydrogen atoms bonded together to make a molecule. Molecules can have huge numbers of atoms, such as or just one atom like Ar.

Per - This means that you divide. If you have 10 apples and 2 people then you divide 10 by 2 to get the number of apples per person. Per always means that something is divided by something else.

Phase - In thermodynamics, usually refers to whether something is gas, liquid, or solid.

Proportional - If something is proportional to something else, then it scales like it. If I say that the cost of going to the movies is proportional to the number of people going, I mean that if 5 people go it costs 5 times more than if 1 person goes.

Vacuum - Having little to no molecules.

The Laws of Motion

Newtonian mechanics contains the laws that govern how objects move around, collide, speed up, or slow down. This post is intended as a review for those who have seen this material before, but maybe don't think about it all the time. We need to know how the pieces move, but we don't need to know a huge amount of detail about it. It might be readable to someone who hasn't ever taken any physics, but it's a lot of information to absorb all at once.

Laws of nature in general become less intimidating when you realize that your brain has specialized hardware to understand them. Our evolutionary ancestors developed this hardware to stay alive, since they needed to judge how fast something was coming, or how much something would hurt if it fell on them, etc. So, all laws of mechanics, and indeed 2 out of 3 laws of thermodynamics, have a "caveman version". This version is correct but incomplete. The caveman version is the part that is innate, but to get the proper understanding you need to think about it with your rational mind.

What we are concerned about is particles. What the hell is a particle? In principle, it could be anything: atoms, footballs, cars, planets, etc. Any thing where the internal motion of its parts doesn't matter can be called a particle as far as we are concerned (for our purposes, we will normally talk about atoms). Particles have mass. They have charge, though most things we talk about are neutral. They have position, which is to say that they are somewhere. They have velocity, which means that they have a speed and are traveling along some direction. Finally, they have acceleration, which is how the speed and/or direction is changing. If something is standing still its velocity is zero. If it's going along with the same speed in the same direction, its acceleration is zero.

Here's the first law of motion:

If there's a rock sitting on the ground, and nothing acts to move it, then it just stays there. Simple. People say something slightly more technical-sounding: an object at rest tends to stay at rest. That's a fine interpretation.

There is also a common addendum to the above: an object in motion tends to stay in motion. This one caveman probably did not know. For it often seems that objects in motion stop on their own, without anyone having touched them. Even a hockey puck on ice will come to a stop sooner or later. To a modern person, it seems natural that you have to keep your foot on the gas to keep your car moving forward. But this is only because he doesn't realize that the gas applied overcomes drag on the car due to air resistance and internal friction. If those forces weren't there, the car would just go on forever, and you'd never have to apply the gas once you got up to speed. Once one realizes that friction and drag are ever-present on Earth, the First law is obvious. However, I assure you that many undergrad college students assert that there is a force of motion that keeps things going. No such thing exists. Things that are moving keep on moving with no forces applied. They only stop if something acts on them.

The full and accurate statement of the First Law is this:

The converse is true. If something does not travel in a straight line with constant speed, then there must be forces on it. Note that being at rest means speed is 0, and so in absence of force speed stays 0, and the caveman form is true. Note also that if something travels in a circle at the same speed, it still has acceleration since its direction is changing (the acceleration is the famous 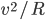centripetal acceleration, which every intro course student is subjected to).

Next up is the Second Law:

Let's unpack this. First, it's not clear what heavy means. If something is heavy, that means that it's hard to lift the object upwards against gravity. And it so happens that things that are hard to lift are also hard to push horizontally, but not always as hard.

This gets at two things at once really. An object has a property—mass—that can't really be explained in terms of other things; it's just mass. And gravity pulls proportionately on massive objects. If something has twice as much mass as another thing, that means it's twice as hard to lift. It also means that it has twice as much friction between it and the ground. And, finally, it means that even if it's on wheels (so that friction is irrelevant), it's twice as hard to get moving. Think of pushing a refrigerator on wheels—just because there's no friction doesn't mean it's a cinch to get going at speed.

In technical terms we would talk about acceleration, which is nothing more than how quickly something picks up speed along a given direction. We say that for a given push 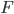, we accelerate an object with mass 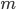by an amount 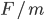along the direction we push. If you push twice as hard, the acceleration is twice as big. If the mass is twice as big, you only get half as much acceleration from the same push.

Of course, what we mean is total force. If an object is acted on from the right and the left equally, these forces cancel so that acceleration is zero. Also, we didn't say what happens if the mass changes. But no matter, the above is good enough for our purposes. So here's a decent statement:

Finally, we have the third law, whose caveman version would be something like "I can punch hard, but when I do my hand hurts!" If something, like a fist, exerts a force on another object (say, Bob's face) then the other object also pushes back with the same force. You can punch a face, but the face also punches your hand with the same force. If you break his face, you might break your hand. In common parlance, for every action there is an equal and opposite reaction.

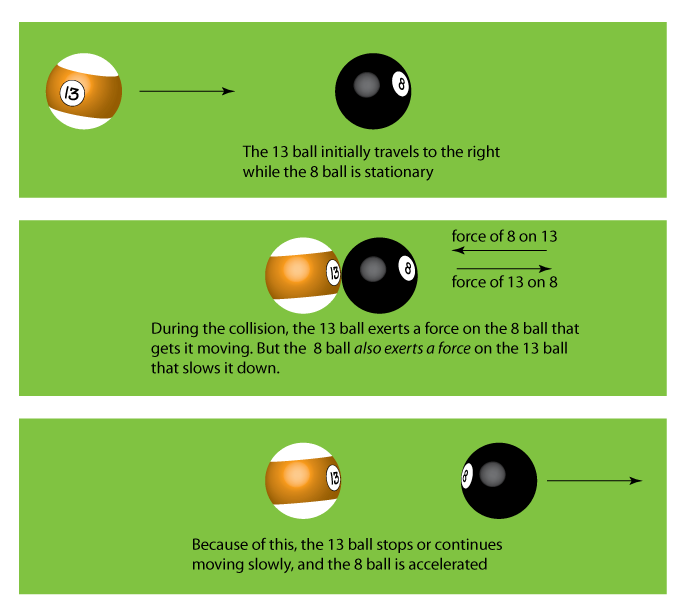
This can be illustrated in the case of a collision in billiards. If the 13 ball is moving to the right, and the 8 ball stationary, then in the collision the 13 ball will push on the 8 ball, and the 8 ball also pushes on the 13 ball in the opposite direction, with exactly the same force.

The above laws are great for getting a feel for real world problems. However, the two conservation laws are, while more abstract, truer than those above, since there is no known exception to either of them (Newton's laws break down quite easily in the quantum world, and the third law is invalidated even without quantum mechanics). I leave those until the next post.